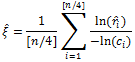
Assume that X is a random variable with a specified distribution rule, described by the function F (X), where X1,…,Xn - is a sample, each element of which is a random value that follows the same distribution rule, as the random value X. Then based on the sample the F (X) function parameters can be estimated.
Assume that the F (X) function belongs to the set of extreme values distribution functions, then to estimate set of parameters (μ, σ, ξ) it is necessary to use method of linear combinations of ratios of spaces.
Consider in details estimation of each parameter:
Estimation of the parameter of the ξ form is based on building a linear combination:
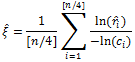
Where:
xq is a selective quantile of the q level.
Layout parameter μ and scale parameter σ are estimated by estimating parameters of a linear regression that looks as y = ax + b, where 
and 
respectively. Regression is built by the n-1 point of the QQ plot (Quantile-Quantile plot) with coordinates:
Where 
is a quantile of extreme values distribution.
See also: