In the probability theory and in mathematical statistics, extreme value (EV) distribution is a set of probability distributions, developed for theory of extreme values. Generalized distribution of extreme values is a generalization of Gumbel, Frechet and Weibull distributions and is used for approximation modeling of maximum of finite sequences of random variables.
Assume that the random variable X is distributed by the rule described by the function:
![]()
Where:
ξ > 0. Form parameter.
![]() . Layout parameter.
. Layout parameter.
![]() . Scale parameter.
. Scale parameter.
Then the random value X has generalized distribution of extreme values or ![]() .
.
Distribution density function:
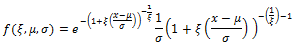
Mean:
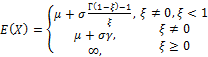
Where γ - Euler constant.
Variance:
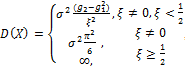
Where ![]() - gamma function.
- gamma function.
Mode:
![]()
Median:
![]()
Kurtosis coefficient:
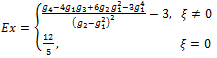
Log-likelihood function for GEV distribution where ξ ≠ 0:
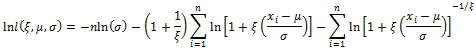
Log-likelihood function for the GEV distribution where ξ = 0:
![]()
To find optimal estimation of parameters it is necessary to maximize both likelihood functions and select the maximum result. This is the best estimation of parameters.
See also:
Library of Methods and Models | ISmGeneralizedExtremeValueDistribution